The Mathematics Of Snowflakes

Summary

The article "The Mathematics Of Snowflakes" explores the intricate relationship between the symmetry, geometry, and fractal nature of snowflakes, and how these properties are governed by the molecular structure of water and the environmental conditions during their formation.

Abstract

Snowflakes are intricate natural phenomena that exhibit hexagonal symmetry due to the molecular structure of water. The article delves into the geometric principles that dictate the formation of snowflakes, emphasizing the tetrahedral shape of water molecules and their arrangement into hexagonal rings and lattices. It explains that the six-fold rotational symmetry and three reflection symmetries of snowflakes are a direct consequence of these geometric patterns. The article also touches on group theory, particularly the D6 group, to classify the diverse symmetries observed in snowflakes and their subgroups. Furthermore, it highlights the fractal properties of snowflakes, drawing parallels with the Koch snowflake as a mathematical representation of their self-similar structures. The text concludes with an invitation for reader engagement and support for the author's work.

Opinions

The author implies that the beauty of snowflakes is deeply rooted in mathematical principles such as symmetry, geometry, and fractals.
The article suggests that understanding the molecular interactions of water is crucial for comprehending the formation and growth of snowflakes.
It is conveyed that group theory provides a robust framework for categorizing the various symmetries present in snowflakes, emphasizing the complexity and diversity of their structures.
The author expresses admiration for the fractal nature of snowflakes, which is elegantly captured by recursive algorithms like the Koch snowflake model.
The author encourages reader interaction by asking for applause and offering a way to support their work through a "buy me a coffee" link, indicating a desire for community engagement and appreciation for their efforts.

A brief explanation of symmetry, geometry, and fractals in snowflakes

Snowflakes are beautiful and complex structures that form when water vapor freezes in the atmosphere. Snowflakes have a hexagonal (six-sided) symmetry, which is determined by the molecular structure and interactions of water. The mathematics behind snowflakes involves geometry, symmetry, and group theory.

Geometry helps us understand how snowflakes form and grow, by describing the shapes and arrangements of the water molecules and the crystals. Water molecules, for example, have a tetrahedral (four-sided pyramid) shape, which allows them to form hexagonal rings when they bond with each other. These rings then stack in a hexagonal lattice, which is the basic unit of a snowflake. As the snowflake falls, it collects more water molecules and forms branches and patterns, which depend on the temperature and humidity of the air.

Symmetry helps us understand how snowflakes look and behave, by describing the invariance and patterns of the crystals. A snowflake has a six-fold rotational symmetry, which means that it looks the same when it is rotated by 60 degrees. A snowflake also has three reflection symmetries, which means that it looks the same when it is flipped over three different axes. These symmetries are related to the hexagonal shape of the water molecules and the crystals.

Group theory is the branch of mathematics that studies the structure and properties of groups, which are sets of elements that can be combined by certain rules.

It helps us understand the diversity and classification of snowflakes, by describing the possible combinations and types of symmetries of the crystals. A snowflake, for instance, belongs to a group called D6, which consists of 12 elements: six rotations and six reflections. Each element of the group represents a transformation that preserves the symmetry of the snowflake.

The group D6 has six subgroups, which represent different types of snowflakes with less symmetry. These subgroups are D3, D2, C6, C3, C2, and C1, where D stands for dihedral (two types of symmetry), C stands for cyclic (one type of symmetry), and the number indicates the order of the group (the number of elements).

Fractal geometry

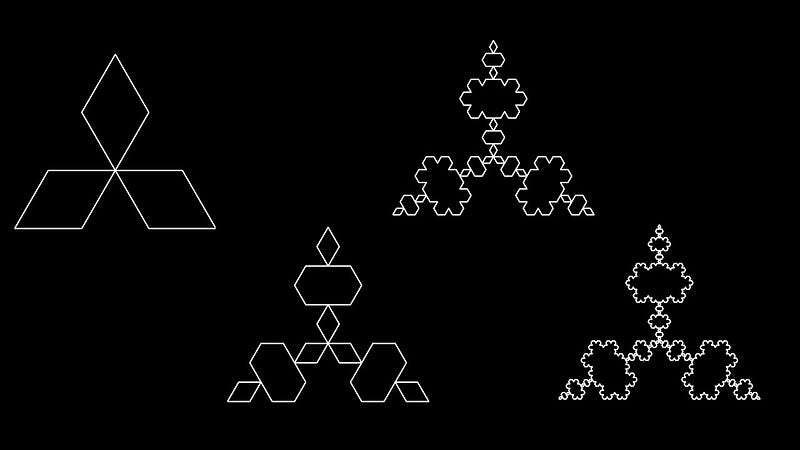
Snowflakes exhibit fractal properties, meaning they have self-similar structures at different scales. The concept of fractals can be described mathematically using recursive algorithms. One famous example is the Koch snowflake, which is a mathematical model that approximates the kind of repeating, self-similar pattern seen in real snowflakes.

Fractal snowflakes. The mathematics is brilliantly explained by Dr. Trefor Bazett

Thank you so much for reading. If you liked this story don’t forget to press that clap icon as many times as you want. If you like my work and want to support me then you can Buy me a coffee ☕️. Keep following for more such stories!